How to learn calculus in 2022 (6 easy steps)

Calculus is the base for advanced math courses, and we use it widely in engineering, scientific research, and technology. Learning and mastering calculus is pertinent to your education and career success.

Most students struggle with calculus because it is very conceptual and application-based. You need to “feel” the calculus concepts via graphs, diagrams and correlate with real-life situations to master them.

In this post, we will discuss how to learn calculus online on your own from scratch, fast and easy. We will discuss several tricks and tips that will help you do so.

Step 1. Master PreCalculus, especially graph plotting

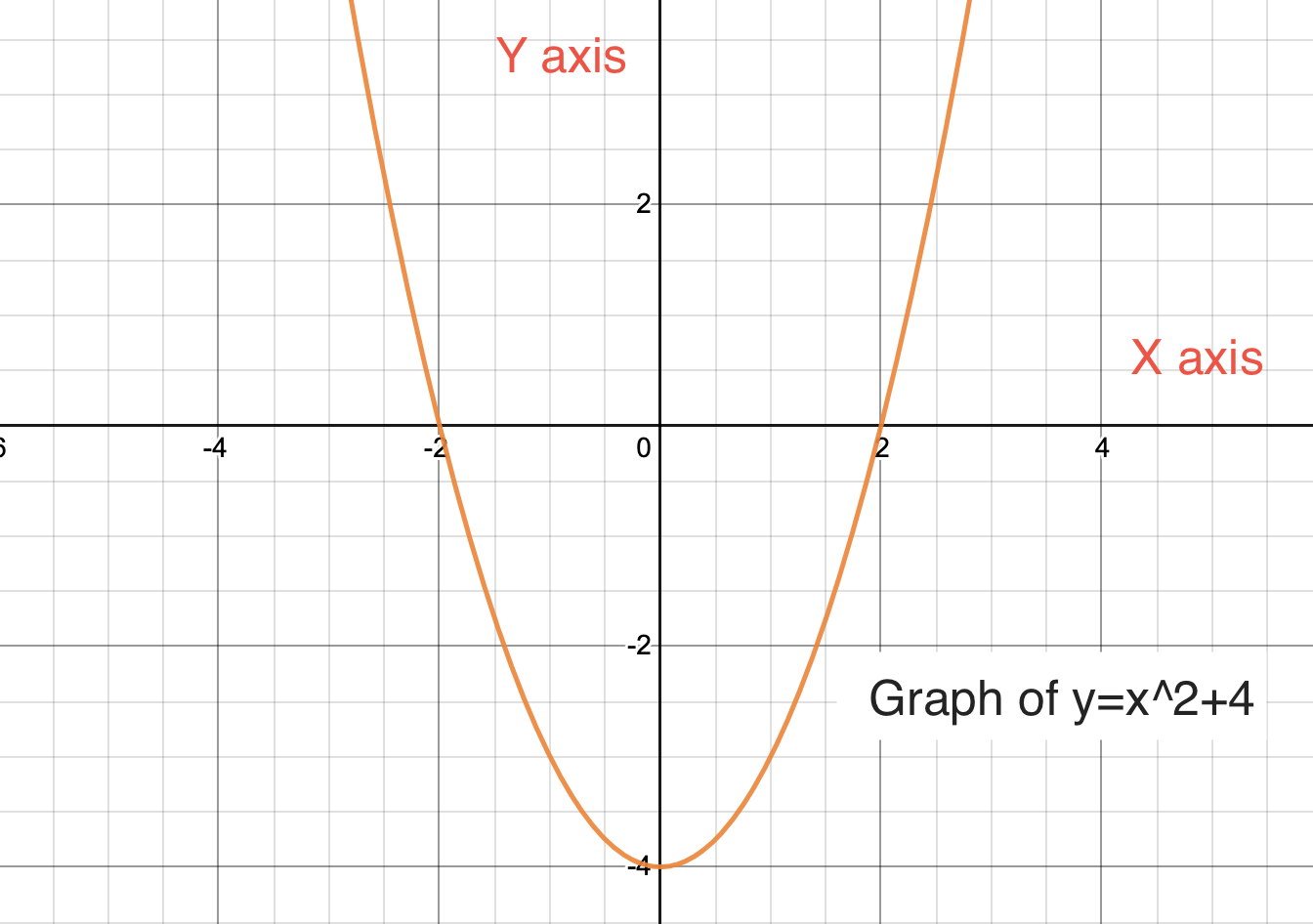
There is a saying, “well begun is half done.” If you want to master calculus, then your base must be strong. You need to master the PreCalculus course to strengthen your base, especially the graph plotting concepts. (Precalculus starts from grade 11th. )

If you don’t know how to plot graphs of various functions, be it algebraic, trigonometric, exponential, or otherwise, you will struggle with even calculus 1, which is the most basic course on calculus 1.

Graph plotting does not mean that you have to take the long way of using 10 to 20 points and plotting each point from scratch. There are several tricks to plot the graph without using a single point. For example, if you already know the graph of y=x^2, which is a concave up parabola, you can easily plot the graph of y+2=x^2 just by shifting the origin. Tricks like this will save enormous time and make you more comfortable in graph plotting. To learn graph plotting tricks in calculus in detail, you can check this video.

Step 2. Graphical interpretation of differentiation

The diagram above gives a graphical interpretation of differentiation. Image credit the math page

We understand that there are other topics like limits, continuity, etc. before differentiation is introduced in calculus. Still, the most important is a graphical interpretation of differentiation (as a measure of rate change). Unless you master this concept, you will not learn the applications of derivatives (especially in complicated situations).

The first derivative (d/dx) is the slope or tangent at a given point on a graph. We also call it tan(θ), where θ is the tangent’s angle with the positive x-axis. This concept is instrumental in solving many geometry-related problems, especially physics and engineering. 

Step 3. Learn applications of integrals

Let us be frank; solving integration problems mathematically has no use in real life. Hence, learning integration techniques though essential is not the ultimate goal of studying integral calculus. We must learn how to apply integration to solve real-life problems. Integration is a technique for the summation of anything. If you want to find the area under a graph bounded by certain lines or other functions, you can use integration techniques. 

Have you ever wondered where the formula S=4*pi*R^2 (surface area of a sphere) came from? You can apply integration techniques learned in calculus 1 to find this formula. You can use it to find the volume of a sphere, the volume of a cone, and the area of an ellipse as well, to name a few.

Step 4. Master Infinite series, convergence and divergence

To learn calculus 2, students struggle the most in Infinite series, convergence, and divergence concepts. For some students, it’s even more complicated than multivariable calculus or calculus 3. 

The only way you can master these concepts is by solving many problems. While solving problems, never get satisfied because you answered them correctly. It would be best to think about why one strategy was better than the other. Was it possible to use any different method to solve the same problem? If yes, would that have been better or worse? Try all possibilities to use the best strategy for similar problems in the future. Also, try to imagine them graphically and see if they make any sense or not. (So basically, you should analyze not only your failures but also your success.)

Step 5. Learn the graphical meaning of differential equations

Did you know that you can solve differential equations graphically too? Many students give more importance to learning higher differential equations mathematically, and they forget the graphical approach completely. Although we can not use the graphical method for all the problems in any meaningful way, mastering its concept is vital to understanding higher-level differential equations. Everything we study is there for a reason, and it is there to solve real-life problems, and if we don’t know about it, our complete understanding of the subject remains shallow at best.

Step 6. Learn graph plotting for 3D functions

The only reason why people find calculus 3 or multivariable calculus hard is because they do not know how to plot graphs in 3D. If we have x,y,z as variables, we can no longer plot the actual graphs on paper. Of course, we can use x,y,z axes to draw it on paper, but it will only be representative and not actual. Many students fail to master 3D graph plotting on paper, and hence they always struggle with calculus 3/multivariable calculus.

We suggest using a 3D plotter to help you understand the 3D graphs. Do so till you learn how to draw it on your own on paper. There is no shortcut, and you have to practice it. The more you practice, the more perfect you will become.

Some FAQs

How long does it take to learn calculus?

Depending on how much time you devote and what you already know, you may need anywhere from 6 months to 3 years to master higher-level calculus. Precalculus may take 2 weeks to 4 months, and Calculus 1, Calculus 2, calculus 3, and differential equations will take the same 3 months to 1 year each. 

Which is the best book on Calculus?

 Stewart calculus, early transcendentals, is undoubtedly the most popular and most recommended book on calculus. 

Summary

So this was all about learning calculus. We covered basic and advanced calculus courses like calculus 1, calculus 2, calculus 3, and differential equations. We mentioned the six most common hurdles that you as a student would face to learn higher-level calculus on your own ( from scratch) and how to cross those hurdles.

My Engineering Buddy team wishes you all the best in your calculus learning. Whether you are on your own or have a tutor, these tips will help you master calculus. It is helpful for both AP Calculus AB, AP calculus BC aspirants and those already studying in colleges and for beginners and advanced students alike.

If you need a private online calculus tutor for yourself, feel free to WhatsApp us, and we will connect you with a top calculus tutor. 

We also provide help in calculus homework. We provide the best online calculus tutoring on the web.